Modeling the Movement of Groundwater VOD in a Rectangular Jumper with a Screen by Bereslavsky EN in Open Access Journal of Biogeneric Science and Research
Abstract
Within the framework of planar steady-state filtration of incompressible fluid according to Darcy's law, an exact analytical solution of the problem of flow in a rectangular cofferdam with a screen in the presence of evaporation from the free surface of groundwater is given. The limiting cases of the considered motion - filtration in unconfined reservoir to imperfect gallery, as well as the flow in the absence of evaporation - are noted.
Introduction
The solution of the problem of fluid inflow to an imperfect well with a flooded filter (i.e. axisymmetric problem) in the exact hydrodynamic formulation is associated with great mathematical difficulties (especially for flows with a free surface) and is not available so far [1-6] (numerous numerical and approximate solutions are not considered here). Therefore, as a first approximation to the solution of this problem, its flat analogues-problems about fluid flow to a rectangular cofferdam with a screen and to an imperfect rectilinear gallery-were considered [1,5-8], which give a certain qualitative insight into the possible dependence of filtration characteristics on the degree of well imperfection. Exact analytical solution of the problem of groundwater movement in unconfined reservoir to imperfect gallery in presence of evaporation from free surface is given in work [9]. As well as an approximate solution of the problem in the case when the flow area on the left is limited by some equipotential defined from the solution. It is shown that the flow pattern near the impermeable screen significantly depends not only on the imperfection of the gallery, but also on the presence of evaporation, which strongly affects the flow rate of the gallery and the ordinate of the exit point of the depression curve on the impermeable wall.
The presented work gives an exact solution of the filtration problem in a rectangular cofferdam with a screen in the presence of evaporation from the free surface of groundwater. In this case, as well as in [9] (unlike in [7, 8] in the area of the flow velocity hodograph appear not rectilinear, but circular polygons, which does not allow using the classical Christoffel-Schwarz formula. The effect of evaporation from the free surface is studied using the method of P.Y. Polubarinova-Kochina [1-6]. Using the methods of conformal mapping of circular polygons developed for special form regions [10-12] [13], the mixed multiparameter boundary value problem of the theory of analytic functions is solved. Taking into account the characteristic features of the flow under consideration makes it possible to obtain the solution through elementary functions, which makes their use the simplest and most convenient. The results of numerical calculations are given and hydrodynamic analysis of the influence of all physical parameters of the model on filtration characteristics is given. Obtained results of plane problem solution give at least some qualitative insight into dependence of flow parameters on degree of well (or tube well) imperfection.
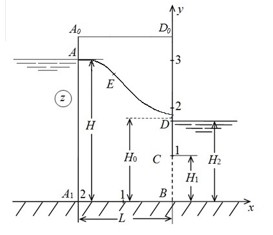
Figure 1: Flow pattern in a rectangular cofferdam with a screen, calculated at ε = 0.5, H = 3, L = 2, H1 = 1,H2 = 1.4.
Material
Figure 1 shows a rectangular cofferdam with slopes A0A1 and D0B on an impermeable horizontal bed of length L. The height of water in the upstream reservoir is H, the downstream reservoir with water level H2, having a partially impermeable vertical wall CD0 (screen), is adjacent to the bottom of the reservoir. If the working part of the cofferdam CB (filter) of width H1 is flooded, i.e. H2 > H1, there is no drawdown gap usual for dams [1]. The upper boundary of the region of motion is the free surface AD overlooking the impermeable screen CD0, from which there is uniform evaporation of intensity ε (0 < ε < 1). The ground is considered homogeneous and isotropic; the fluid flow obeys the Darcy law with known filtration coefficient κ = const.
Let us introduce a complex potential of motion ω= φ+iψ, where the velocity potential, the current function and the complex coordinate are referred to kNiH, where Ni is the head at point A. At the choice of the coordinate system indicated in (Figure 1) and coincidence of the head comparison plane with the plane y=0 at the boundary of the filtration region, the following boundary conditions are fulfilled:

The task is to determine the position of the free surface AD and to find the ordinate H0 of the exit point of the depression curve on the screen, as well as the filtration flow rate Q.
Method
To solve the problem we use the method of P.Ya. Polubarinova-Kochina, which is based on the application of the analytic theory of the Fuchs class linear differential equations [1, 6, 14]. We introduce an auxiliary canonical variable ζ and functions: z(ζ) conformally mapping the upper half-plane ζ>0 to the flow region z under the correspondence of points ζD=0, ζE=e, ζA=1, ζA1=a1, ζB=b, (a1,b are unknown affixes of points A1 and B in the plane ζ),ζC=∞, and functions dω / dζ and dz / dζ. We emphasize that, compared to [9], an additional boundary angular singular point A1 appears here in the flow region z, which complicates the solution considerably.
By determining the characteristic indices of the functions dω / dζ and dz / dζ near regular singular points [1, 6, 14], we find that they are linear combinations of two branches of the following Riemann function [1, 6, 14]:

where . The last Riemann symbol corresponds to the following Fuchs class linear differential equation with four regular singular points:

It is well known [1-6, 14] that difficulties of principle character arise during integration of equations of this kind. They are caused by the fact that the coefficients of equation (3) besides the uncertain affixe also contain an additional, so called accessory parameter λ, also unknown beforehand, and so far there is no effective way of their actual finding.
Let us turn to the region of the complex velocity w corresponding to the boundary conditions (1), which is depicted in (Figure 2). This region, which is a circular quadrilateral ABCDE with a cut with a vertex at point E (corresponding to the inflection point of the depression curve) and an angle νπ at the vertex A, belongs to the class of circular polygons in polar meshes and was studied earlier [13]. It is important to emphasize that such areas, despite their particular form, however, are very typical and typical for many problems of underground hydromechanics: in filtration from canals, irrigators and reservoirs, in freshwater currents over resting saline waters, in problems of Zhukovsky sheet flowing in presence of saline retaining waters (see, for example, [11-15].
Replacing the variables ζ=th2t translates the upper half-plane ζ into the horizontal half-plane Re t>0, 0< Im t<0.5π of the parametric plane t in agreement with the points tA=∞, tD=0, tC=0.5π, tB=arcth +0.5π, tA1=arcth +0.5π (1< a1< b< ∞), and the Y integrals of equation (3), which were constructed by the method [13], transforms to the form

where C(C≠1) is an unknown fitting constant.
Taking into account relation (2) and considering that w =dω / dz, we arrive at the dependencies we are looking for

where M>0 is the scale constant of the simulation.
One can check that the functions (5) satisfy the boundary conditions (1) reformulated in terms of the functions dω / dti dz / dt and, thus, are the parametric solution of the original boundary value problem. Writing representations (5) for different parts of the half-belt boundary followed by integration over the whole contour of the area of the parametric variable t leads to the closure of the flow area and, thus, serves to control the calculations.
Result. As a result, we get expressions for the following values: the width L of the cofferdam, the water levels in the upper H and lower H2 pools, and the length H1 of the filter

of the required coordinates of the free surface points AD

and expressions for the filtration flow rate Q and the free surface exit point ordinate
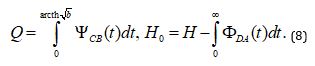
Other expressions for Q,H0 and L are used to control the calculations:


as well as the expression

directly derived from the boundary conditions (1).
In formulas (5) - (10), the integrand functions are expressions of the right-hand sides of equations (3) on the corresponding parts of the contour of the auxiliary region t.
Limit cases.
- At L→∞, i.e. at the junction of points A1 and A, in plane t, i.e. at a1→1 ( arcth a1 = ∞), the cofferdam degenerates into a semi-infinite left-handed unconfined formation. Thus, the exact solution of groundwater flow to the imperfect gallery, studied earlier [9], is obtained.
- At ԑ→0, i.e. at small values of evaporation intensity the results of works [7, 8] are obtained.
Discussion
Representations (5) - (10) contain four unknown constants M, C, a1 and b. The parameters a1, b (1< a1 < b < ∞), C (C 1) are determined from equations (6) for the given values H1, H2 (H1 ≤ H2 < H) and L, while the simulation constant M is found from the second equation (6) fixing the water level H in the headwater of the cofferdam. After determination of the unknown constants the filtration flow rate Q and the ordinate H0 of the outlet point of the depression curve on the impermeable section DC by formulas (8) and coordinates of points of free surface DA by formulas (7) are sequentially found.
Table 1: Results of calculations of Q and H0 values when varying ε, H and L.

Table 2: Results of calculations of Q and H0 values when varying H1 and H2.


Figure 2: Area of complex velocity w.

Figure 3: Dependence of the cofferdam flow rate Q and the ordinate H0 of the free surface outlet point H0 on the filter length H1.

Figure 4: Dependence of the cofferdam flow rate Q and the ordinate H0 of the free surface outlet point on the water level in the downstream reservoir H2.
Figure 1 shows the flow pattern calculated at ε = 0. 5, H = 3, L = 2, H1 = 1.0, H2 = 1.4 (base case [9]). Results of calculations of influence of defining physical parameters ε, H, H1, H2 and L on values Q and H0 are presented in (Tables 1 & 2). On (Figures 3 & 4) dependences of flow Q (curves 1) and ordinate H0 of a point of an exit of a depression curve on a screen (curves 2) from parameters H1 and H2 are submitted. Analysis of calculations of these tables and graphs allows us to draw the following conclusions:
- Decrease of intensity of evaporation ε and increase of head H accompany increase of flow Q and ordinate H0 of the exit point of depression curve on the screen;
- Decrease of the screen depth H1 and increase of the water level in the downstream H2 are accompanied by a decrease of the flow Q and increase of the ordinate H0;
- As the width of the cofferdam L increases, the flow rate Q and the ordinate H0 of the free surface exit point to the screen decrease.
From (Table 2) and (Figures 3 & 4) follows that decrease of parameters H1 and H2 by 1.5 and 1.3 times, respectively, leads to change of Q value by 16.8 % (at fixing H1) and 12 % (at fixing H2). The marked regularities lead to the conclusion that the cofferdam flow rate depends on the value of level lowering to a somewhat greater extent than on the filter length (or on imperfection of a well or a well).
For the base case, almost all the dependences of Q and H0 on the parameters ε, H, H1, H2, and L are close to linear. Comparison of the exact values obtained for the base case Q =1.155 and H0=1.776 with the approximate values Q =1.141 and H0=1.768 for the base case [9] where the flow area to the left was limited by the equipotential shows that the relative error of the calculations is rather small and amounts to only 0.5 and 1.3% respectively.
Comparison of exact value of flow Q =1.16, obtained for basic variant, with approximate value Q =1.26, which follows at application of generalized formula of I.A. Charnyi [1, p. 267] for usual rectangular cofferdam (without screen) in presence of evaporation

leads to an error of 8.3%.
For comparison with data H=1, H1=0.05, H2=0.238, L=4 work [7] at absence of evaporation, i.e. at ε =0, for which values Q=0.118, H0=0.29 are received by the approximate formulas in semi-inverse formulation, we consider variant ε =0.1, H=1, H1=0.05, H2=0.238, L=4, leading to exact values Q=0.42, H0=0.75. Here, relative calculation errors are 71 and 61%, respectively. Consequently, just as in [9], evaporation significantly affects the flow pattern.
Conclusion
The method for construction of exact analytical solution of a problem about movement of liquid in a rectangular cofferdam with a screen in the presence of evaporation from a free surface of ground waters has been developed. The investigation shows that the filtration scheme in a rectangular cofferdam with impermeable screen, firstly, is very similar to the previously considered [9] problem about movement of ground waters to the imperfect gallery, one of them being limiting with respect to the other. Secondly, the flow pattern near the screen essentially depends not only on the filter size, but also on the presence of evaporation, which strongly affects the flow rate value and the ordinate of the outlet point of the depression curve on the screen. The obtained results, announced in [16], give some idea (at least qualitatively) about possible dependence of motion characteristics when considering the filtration problem already to imperfect well or tubular well.
More information regarding this Article visit: OAJBGSR

No comments:
Post a Comment